BY LETTER
Lagrange Points
In any system with one large and one small orbiting body (e.g. a planet and a moon, star and a planet, or a double planet or binary star), a set of five points where a still smaller body (e.g. a ship or habitat or smaller moon) would retain a fixed position with respect to the other two.
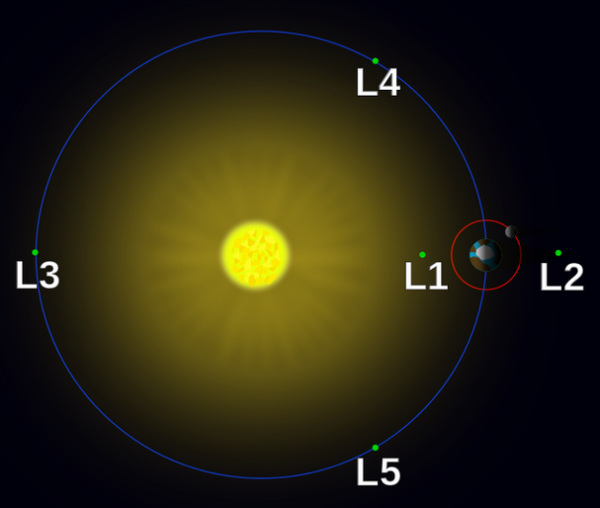
The Lagrange points are a set of five points that occur between two large objects, the gravitational force of the large objects is exactly equal to the centripetal force required to rotate with the objects (mathematically, these are libration solutions to the three body problem). At these points, a small orbiting object can orbit at a constant distance from both of the larger masses.
L1 - the point between the two bodies where the gravitational attractions negate each other ( in the case of CisLunar space - 325,000 kilometres from the Earth and 56,000 kilometres from the Moon).
L2 the point on the far side of one of the bodies, where the component forces combine (in CisLunar space - 447,000 kilometres from the Earth and 67,000 kilometres from the Moon).
L3 - a point in one of the bodies orbit directly opposite it (in CisLunar space - 380,000 kilometres from the Earth and 760,000 kilometres from the Moon).
These three points (L1, L2, and L3) are unstable; they are saddle-shaped gravitational valleys in which a body displaced perpendicular to the axis of two large objects is drawn back toward the axis. Since displacement along the axis can continue indefinitely, these as known as points of unstable equilibrium.
L4 - one of the two stable Lagrange points that forms an equilateral triangle with the two large masses. In CisLunar space this is the point 60° east (i.e., the direction of the Earth's rotation or counterclockwise) of the Moon, leading it in orbit around the Earth
L5 - the other stable Lagrange point. In CisLunar space is the point 60° west (clockwise) of the Moon, trailing it in orbit around the Earth.
L4 and L5 are each bowl-shaped gravitational valleys in which a body displaced in any direction returns to the centre and are therefore known as points of stable equilibrium. These are also known as Trojan points
The Lagrange points are named for the first Terragen to describe them, the Old Earth Industrial Age Italian-French mathematician Joseph-Louis Lagrange who lived from 233-156 BT (1736-1813 c.e.). Lagrange's theories were confirmed a century later with the discovery of the Trojan asteroids in the orbit of Jupiter in 63 BT (1906 c.e.), exactly where L4 and L5 would have been had Jupiter been the Moon and Sol been the Earth. The discovery was so profound at the time that, to this day, the L4 and L5 points in any orbit are referred to by astronomers as the leading or trailing Trojan points.
Lagrangian libration points are critical to the construction of orbital space habitats because they provide a stable point for large objects, which will continue to hold station with little expenditure of necessary propulsive mass for expensive course corrections. They also occupy an optimal position from their parent bodies in terms of both distance and velocity change (deltaV). Since the majority of velocity change (about 8.6 kilometres per second or two thirds of a total 12.7 kilometres per second deltaV) is expended in reaching low orbit, one can travel to or between any of the Lagrange colonies with equal ease. For these two reasons they are highly prized by space engineers and development corporations.
Related Articles
- Lagrange Defenders
- Lagrange Magshield, The
- Lagrange Snow - Text by Anders Sandberg
Traditional Sol System dessert/confection, invented by the early space colonists. It consists of cream (bovine or from soy) and egg whites whipped together with flavoring and then exposed to a minute of vacuum and sunlight. The vacuum causes air bubbles to expand, while the ultraviolet radiation denatures the protein into an elastic matrix. The result is an ultra-light, foamy dessert usually served immediately with fruits. Due to its instability it cannot be stored. - Lyaponov Tubes
Appears in Topics
Development Notes
Text by M. Alan Kazlev
Initially published on 03 December 2001.
Initially published on 03 December 2001.